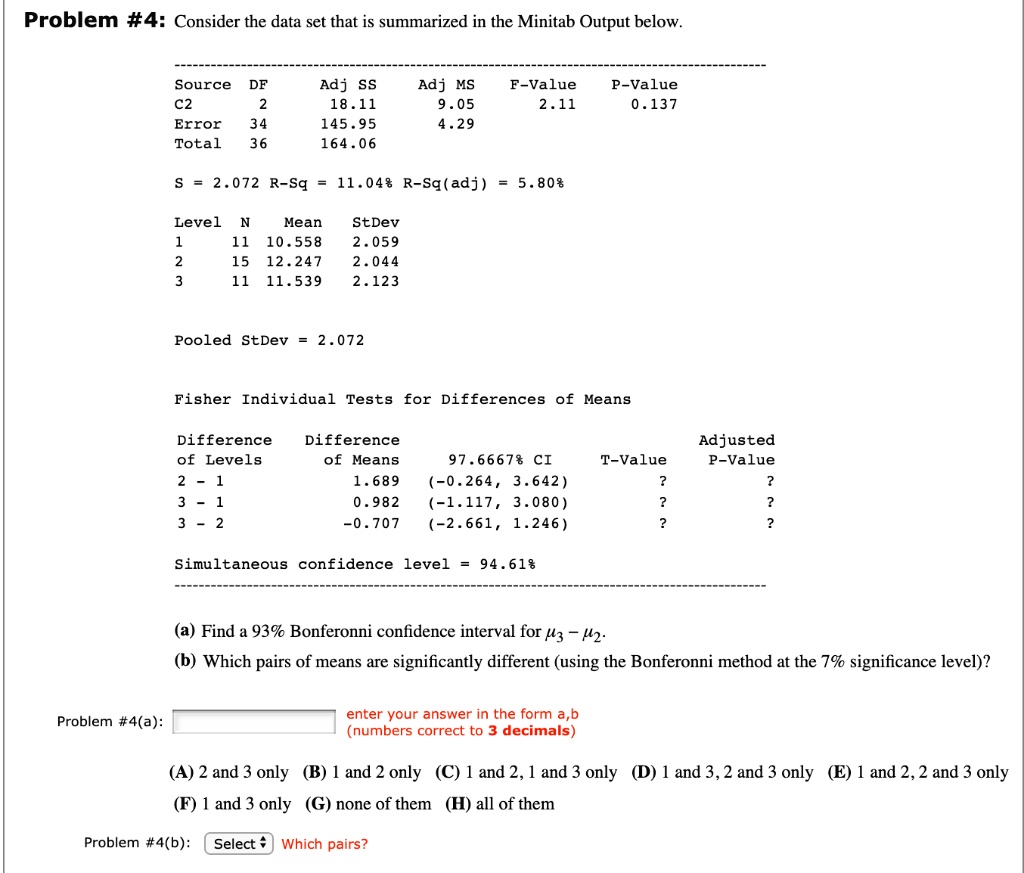
What is the equation of your regression line? Based on your scatterplot and residual plot, does linear regression seem like an appropriate way to predict heights?
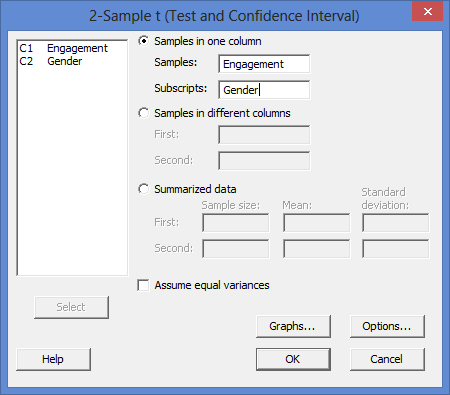
Begin by using linear regression to predict a child's height at age 9 from the child's height at age 2. In the Minitab output, under "Predicted Values for New Observations", will be the x-value that you typed in (under "Obs"), the predicted value for the y-variable (under "Fit"), a 95 percent confidence interval for the mean response for this value of the explanatory variable (under "95% CI"), and a 95 percent prediction interval for an individual response at this value of the explanatory variable (under "95% PI"). You can also adjust the confidence level if necessary. Then you can check "confidence limits" and/or "prediction limits" below. This should be the value of the x-variable for which you want to obtain a confidence interval or prediction interval for the response. Type a value for the x-variable in the box "Prediction intervals for new observations". To obtain these intervals, go to Stat -> Regression -> Regression, choose the response and predictor variables as usual, and then click on the "Options" button. Minitab will also calculate confidence intervals for the mean response and prediction intervals for an individual response, given a particular value for the explanatory variable. You can also get a histogram or a normal probability plot of the residuals by checking the appropriate boxes. To make sure you get a residual plot along with your regression, click on "Graphs" and the put the explanatory variable in the box under "Residuals versus the variables". The table of output that you get includes not only the regression coefficients but also the information that you need to do inference for the regression slope: the standard errors for the estimated coefficients, and the t-statistic and p-value for the test that the slope is zero against a two-sided alternative. Recall that you can make scatterplots by going to Graph -> ScatterplotĪnd carry out linear regression by going to Stat -> Regression -> Regression. Height of the child in centimeters at age 18 Weight of the child in kilograms at age 18 Height of the child in centimeters at age 9 Weight of the child in kilograms at age 9 Height of the child in centimeters at age 2 Weight of the child in kilograms at age 2 The results of the original study were published in. These children were measured at ages 2, 9, and 18. The study involved 136 children, all born in Berkeley, CA in 1928-1929. The data come from the Berkeley guidance study of children and were found here. #HOW TO DO ONE MEAN CONFIDENCE INTERVALS ON MINITAB 18 HOW TO#
You will determine how to predict a child's height or weight at a later age from their height or weight at an earlier age.įirst, open the data set GROWTH, which is available in TED.
In this lab, you will investigate how fast children grow. Math 11L, Lab 8 Lab 8: Predicting children's growth (Regression inference)
0 kommentar(er)
